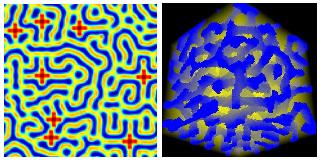
Turing patterns:

In the 1950s British mathematician Alan Turing proposed a simple reaction-diffusion system describing chemical reactions and diffusion to account for morphogenesis, i.e., the development of form and shape in biological systems. Due to the complexity of nature, researchers have not yet succeeded in developing a Turing system based model that would describe morphogenesis in essence, although specific examples such as the skin coloring of animals have been modeled using Turing systems.

Turing systems show a very rich behavior from the pattern formation point of view, which means that by numerically solving these mathematically defined systems we obtain a variety of spatial patterns in two dimensions and structures in three dimensions, varying from spots to stripes and from lamellar to chaotic structures. In this study we have obtained results for a three-dimensional system, which, to the authors' knowledge, has never before been studied using numerical simulations. We have also studied the basic characteristics of a three-dimensional Turing system and compared them with those of a two-dimensional one. In 3D the morphological development becomes more interesting and complex. We have also studied the transition between two- and three-dimensional morphologies. In addition, the robustness of Turing structures has been investigated against Gaussian random noise.

Our motivation for studying the Turing systems is biological. The figures show how connections between certain points can be grown by using a Turing system with sources of chemicals. The resulting connected network has many interesting properties, and in the future work we will concentrate on a Turing system and an active random walker model combined to explain some of the features of neural patterning, i.e., how neurons establish connections to other neurons.

  

Figure: Complex networks generated by Turing system in two and three dimensions.

Our publications related to Turing systems

• A new dimension to Turing patterns,
  T. Leppänen, M. Karttunen, K. Kaski, R. A. Barrio, and L. Zhang,
  Physica D 168-169C, 35-44 (2002).
  [Online]

• The effect of noise on Turing patterns,
  T. Leppänen, M. Karttunen, K. Kaski, and R. A. Barrio,
  Prog. Theor. Phys. (Suppl.) 150, 367-370 (2003).
  [Online]

• Dimensionality effects in Turing pattern formation
  T. Leppänen, M. Karttunen, K. Kaski, and R. A. Barrio
  Int. J. Mod. Phys. B 17, 5541-5553 (2003).
  [Preprint]

• Turing systems as models of complex pattern formation
  T. Leppänen, M. Karttunen, K. Kaski, and R. A. Barrio,
  Braz. J. Phys. 34, 368-372 (2004).
  [Preprint]

• Morphological transitions and bistability in Turing systems
  T. Leppänen, M. Karttunen, R.A. Barrio, and K. Kaski
  Phys. Rev. E 70, 066202 (2004).
  [Preprint]

• The theory of Turing pattern formation
  T. Leppänen
  to appear in Current Topics in Physics (Imperial College Press), 2004.
  [Preprint]

• Spatio-temporal dynamics in a Turing model
  T. Leppänen, M. Karttunen, R.A. Barrio, and K. Kaski
  submitted to InterJournal, 2004.
  [Preprint]

• More: see our publication list.

Movies of spatio-temporal pattern formation:

• Turing-Hopf bifurcation in a monostable system
• Turing-Hopf-Turing bifurcation in a tristable system with twinkling spots
• Turing-Turing bifurcation (coupled with Hopf) in a tristable system

• The self-organization of spherical structures in 3D
• The self-organization of spherical structures under Gaussian noise in 3D
• The self-organization of spherical structures under strong Gaussian noise in 3D
• The self-organization of a network with Gaussian noise in 2D
• The self-organization of a network in 3D
• Self-assembly of a planar lamellae in 3D
• Self-assembly of a minimal surface in the presence of noise in 3D
• Time dependent oscillations in 3D under noise